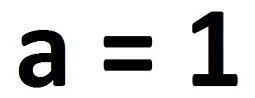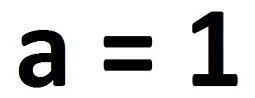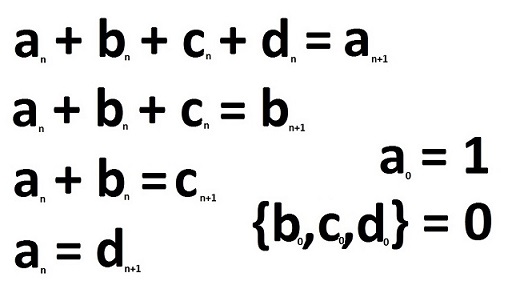Infinite Range of Golden Ratios
Infinite PHI – ∞ φ
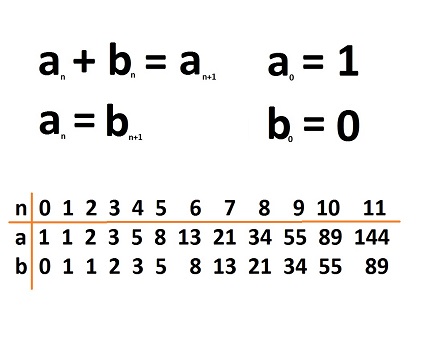
PHI series of numbers, 1st order: 1
Since this is a 1st order PHI, it has no further iterations. Once is all unity needs to authenticate itself.
It also has no relationship with any other value, so there is no need to approximate its solution by dividing some other number into unity!
Hence, all we get is...
X = 1
Subtracting 1 from both sides, we get...
A linear polynomial in one unknown: X − 1 = 0
PHI series of numbers, 2nd order:
|
a
|
|
b
|
|
|---|
|
1
|
÷
|
0
|
=
|
NULL
|
|
1
|
÷
|
1
|
=
|
1
|
|
2
|
÷
|
1
|
=
|
2
|
|
3
|
÷
|
2
|
=
|
1.5
|
|
5
|
÷
|
3
|
=
|
1.6666666666667
|
|
8
|
÷
|
5
|
=
|
1.6
|
|
13
|
÷
|
8
|
=
|
1.625
|
|
21
|
÷
|
13
|
=
|
1.6153846153846
|
|
34
|
÷
|
21
|
=
|
1.6190476190476
|
|
55
|
÷
|
34
|
=
|
1.6176470588235
|
|
89
|
÷
|
55
|
=
|
1.6181818181818
|
|
144
|
÷
|
89
|
=
|
1.6179775280899
|
|
233
|
÷
|
144
|
=
|
1.6180555555556
|
|
377
|
÷
|
233
|
=
|
1.618025751073
|
|
610
|
÷
|
377
|
=
|
1.6180371352785
|
|
987
|
÷
|
610
|
=
|
1.6180327868852
|
|
1597
|
÷
|
987
|
=
|
1.6180344478217
|
|
2584
|
÷
|
1597
|
=
|
1.6180338134001
|
|
4181
|
÷
|
2584
|
=
|
1.6180340557276
|
|
6765
|
÷
|
4181
|
=
|
1.6180339631667
|
|
10946
|
÷
|
6765
|
=
|
1.6180339985218
|
|
17711
|
÷
|
10946
|
=
|
1.6180339850174
|
|
28657
|
÷
|
17711
|
=
|
1.6180339901756
|
|
46368
|
÷
|
28657
|
=
|
1.6180339882053
|
|
75025
|
÷
|
46368
|
=
|
1.6180339889579
|
|
121393
|
÷
|
75025
|
=
|
1.6180339886704
|
|
196418
|
÷
|
121393
|
=
|
1.6180339887802
|
|
317811
|
÷
|
196418
|
=
|
1.6180339887383
|
|
514229
|
÷
|
317811
|
=
|
1.6180339887543
|
|
832040
|
÷
|
514229
|
=
|
1.6180339887482
|
|
1346269
|
÷
|
832040
|
=
|
1.6180339887505
|
|
2178309
|
÷
|
1346269
|
=
|
1.6180339887496
|
|
3524578
|
÷
|
2178309
|
=
|
1.61803398875
|
|
5702887
|
÷
|
3524578
|
=
|
1.6180339887499
|
|
9227465
|
÷
|
5702887
|
=
|
1.6180339887499
|
|
14930352
|
÷
|
9227465
|
=
|
1.6180339887499
|
|
|
After 35 iterations, it's pretty obvious that the approximation of the 2nd order of PHI accurate to eleven decimal places is...
1.6180339887499
And it's reciprocal is...
0.6180339887499
To be able to make a 2nd order polynomial in one unknown out of these two numbers will require that one of them is given a negative sign value...
− 0.6180339887499
Now we can form this polynomial by multiplying these two values together. But first, we have to turn them into linear expressions in one unknown...
X1 = 1.6180339887499
Subtract 1.6180339887499 from both sides of the equal sign...
X1 − 1.6180339887499 = 1.6180339887499 − 1.6180339887499
Yields...
(X − 1.6180339887499) = 0
X2 = − 0.6180339887499
Add 0.6180339887499 to both sides of the equal sign...
X2 + 0.6180339887499 = − 0.6180339887499 + 0.6180339887499
Yields...
(X + 0.6180339887499) = 0
Multiplying these two roots together...
(X − 1.6180339887499) × (X + 0.6180339887499) = 0
Yields...
X2 + 0.6180339887499X1 − 1.6180339887499X1 − 1 = 0
Simplifying further, yields a quadratic polynomial in one unknown...
X2 − X1 − 1 = 0
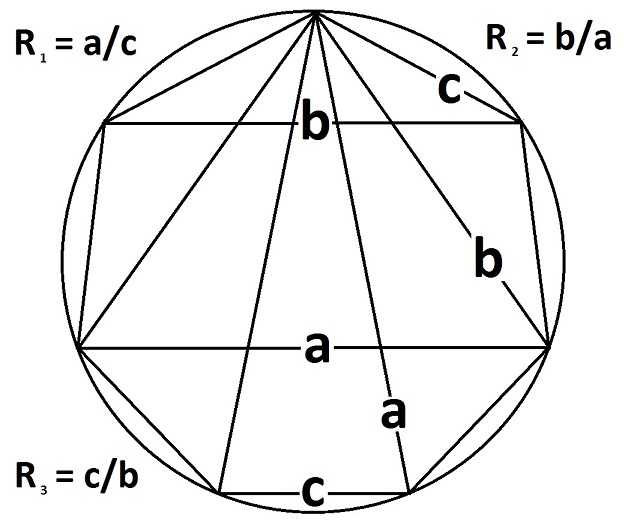
PHI series of numbers, 3rd order:

|
|
|
|
a
|
b
|
c
|
|---|
|
1
|
0
|
0
|
|
1
|
1
|
1
|
|
3
|
2
|
1
|
|
6
|
5
|
3
|
|
14
|
11
|
6
|
|
31
|
25
|
14
|
|
70
|
56
|
31
|
|
157
|
126
|
70
|
|
353
|
283
|
157
|
|
793
|
636
|
353
|
|
1782
|
1429
|
793
|
|
4004
|
3211
|
1782
|
|
8997
|
7215
|
4004
|
|
20216
|
16212
|
8997
|
|
45425
|
36428
|
20216
|
|
102069
|
81853
|
45425
|
|
229347
|
183922
|
102069
|
|
515338
|
413269
|
229347
|
|
1157954
|
928607
|
515338
|
|
2601899
|
2086561
|
1157954
|
|
5846414
|
4688460
|
2601899
|
|
13136773
|
10534874
|
5846414
|
|
29518061
|
23671647
|
13136773
|
|
66326481
|
53189708
|
29518061
|
|
149034250
|
119516189
|
66326481
|
|
334876920
|
268550439
|
149034250
|
|
752461609
|
603427359
|
334876920
|
|
1690765888
|
1355888968
|
752461609
|
|
3799116465
|
3046654856
|
1690765888
|
|
8536537209
|
6845771321
|
3799116465
|
|
19181424995
|
15382308530
|
8536537209
|
|
43100270734
|
34563733525
|
19181424995
|
|
96845429254
|
77664004259
|
43100270734
|
|
217609704247
|
174509433513
|
96845429254
|
|
488964567014
|
392119137760
|
217609704247
|
|
1098693409021
|
881083704774
|
488964567014
|
|
2468741680809
|
1979777113795
|
1098693409021
|
|
5547212203625
|
4448518794604
|
2468741680809
|
|
12464472679038
|
9995730998229
|
5547212203625
|
|
28007415880892
|
22460203677267
|
12464472679038
|
|
62932092237197
|
50467619558159
|
28007415880892
|
|
141407127676248
|
113399711795356
|
62932092237197
|
|
317738931708801
|
254806839471604
|
141407127676248
|
|
713952898856653
|
572545771180405
|
317738931708801
|
|
1604237601745859
|
1286498670037058
|
713952898856653
|
|
3604689170639570
|
2890736271782917
|
1604237601745859
|
|
8099663044168346
|
6495425442422487
|
3604689170639570
|
|
18199777657230403
|
14595088486590833
|
8099663044168346
|
|
40894529187989582
|
32794866143821236
|
18199777657230403
|
|
91889172989041221
|
73689395331810818
|
40894529187989582
|
|
206473097508841621
|
165578568320852039
|
91889172989041221
|
|
463940838818734881
|
372051665829693660
|
206473097508841621
|
|
1042465602157270162
|
835992504648428541
|
463940838818734881
|
|
2342398945624433584
|
1878458106805698703
|
1042465602157270162
|
|
5263322654587402449
|
4220857052430132287
|
2342398945624433584
|
After 54 iterations, the approximation of the three roots of the 2nd order of PHI accurate to eleven decimal places are...
X1 = a/c = 5263322654587402449 ÷ 2342398945624433584 = 2.2469796037175
X2 = b/a = 4220857052430132287 ÷ 5263322654587402449 = 0.80193773580484
X3 = c/b = 2342398945624433584 ÷ 4220857052430132287 = 0.55495813208737
To be able to make a 3rd order polynomial in one unknown out of these three numbers will require that one of them is given a negative sign value...
− 0.80193773580484
Now we can form this polynomial by multiplying these three values together. But first, we have to turn them into linear expressions in one unknown...
X1 = 2.2469796037175
Subtract 2.2469796037175 from both sides of the equal sign...
X1 − 2.2469796037175 = 2.2469796037175 − 2.2469796037175
Yields...
(X − 2.2469796037175) = 0
X2 = − 0.80193773580484
Add 0.80193773580484 to both sides of the equal sign...
X2 + 0.80193773580484 = − 0.80193773580484 + 0.80193773580484
Yields...
(X + 0.80193773580484) = 0
X3 = 0.55495813208737
Subtract 0.55495813208737 from both sides of the equal sign...
X3 − 0.55495813208737 = 0.55495813208737 − 0.55495813208737
Yields...
(X − 0.55495813208737) = 0
Multiplying these three roots together...
(X − 2.2469796037175) × (X + 0.80193773580484) × (X − 0.55495813208737) = 0
Yields...
X3 − 2.2469796037175X2 + 0.80193773580484X2 − 0.55495813208737X2 − 1.8019377358048X1 + 1.2469796037175X1 − 0.44504186791263X1 + 1 = 0
Simplifying further, yields a 3rd order polynomial in one unknown...
X3 -2X2 -1X1 + 1 = 0
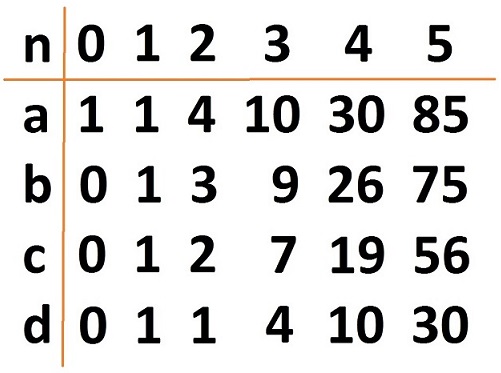
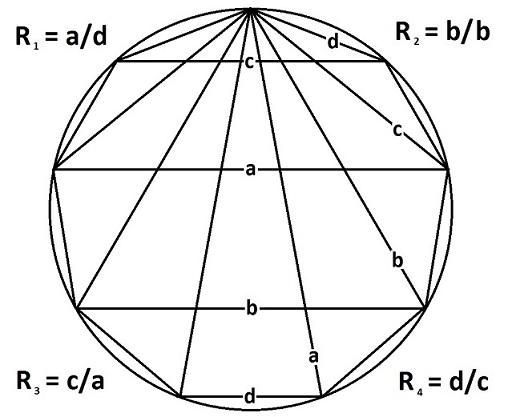
PHI series of numbers, 4th order:
|
a
|
b
|
c
|
d
|
|---|
|
1
|
0
|
0
|
0
|
|
1
|
1
|
1
|
1
|
|
4
|
3
|
2
|
1
|
|
10
|
9
|
7
|
4
|
|
30
|
26
|
19
|
10
|
|
85
|
75
|
56
|
30
|
|
246
|
216
|
160
|
85
|
|
707
|
622
|
462
|
246
|
|
2037
|
1791
|
1329
|
707
|
|
5864
|
5157
|
3828
|
2037
|
|
16886
|
14849
|
11021
|
5864
|
|
48620
|
42756
|
31735
|
16886
|
|
139997
|
123111
|
91376
|
48620
|
|
403104
|
354484
|
263108
|
139997
|
|
1160693
|
1020696
|
757588
|
403104
|
|
3342081
|
2938977
|
2181389
|
1160693
|
|
9623140
|
8462447
|
6281058
|
3342081
|
|
27708726
|
24366645
|
18085587
|
9623140
|
|
79784098
|
70160958
|
52075371
|
27708726
|
|
229729153
|
202020427
|
149945056
|
79784098
|
|
661478734
|
581694636
|
431749580
|
229729153
|
|
1904652103
|
1674922950
|
1243173370
|
661478734
|
|
5484227157
|
4822748423
|
3579575053
|
1904652103
|
|
15791202736
|
13886550633
|
10306975580
|
5484227157
|
|
45468956106
|
39984728949
|
29677753369
|
15791202736
|
|
130922641160
|
115131438424
|
85453685055
|
45468956106
|
|
376976720745
|
331507764639
|
246054079584
|
130922641160
|
|
1085461206128
|
954538564968
|
708484485384
|
376976720745
|
|
3125460977225
|
2748484256480
|
2039999771096
|
1085461206128
|
|
8999406210929
|
7913945004801
|
5873945233705
|
3125460977225
|
|
25912757426660
|
22787296449435
|
16913351215730
|
8999406210929
|
|
74612811302754
|
65613405091825
|
48700053876095
|
25912757426660
|
|
214839027697334
|
188926270270674
|
140226216394579
|
74612811302754
|
|
618604325665341
|
543991514362587
|
403765297968008
|
214839027697334
|
|
1781200165693270
|
1566361137995936
|
1162595840027928
|
618604325665341
|
|
5128761469382475
|
4510157143717134
|
3347561303689206
|
1781200165693270
|
|
14767680082482085
|
12986479916788815
|
9638918613099609
|
5128761469382475
|
|
42521840081752984
|
37393078612370509
|
27754159999270900
|
14767680082482085
|
|
122436758775876478
|
107669078693394393
|
79914918694123493
|
42521840081752984
|
|
352542596245147348
|
310020756163394364
|
230105837469270871
|
122436758775876478
|
|
1015105948653689061
|
892669189877812583
|
662563352408541712
|
352542596245147348
|
|
2922881087185190704
|
2570338490940043356
|
1907775138531501644
|
1015105948653689061
|
|
8416100665310424765
|
7400994716656735704
|
5493219578125234060
|
2922881087185190704
|
After 42 iterations, the approximation of the four roots of the 4th order of PHI accurate to eleven decimal places are...
X1 = a/d = 8416100665310424765 ÷ 2922881087185190704 = 2.8793852415718
X2 = b/b = 7400994716656735704 ÷ 7400994716656735704 = 1
X3 = c/a = 5493219578125234060 ÷ 8416100665310424765 = 0.65270364466614
X4 = d/c = 2922881087185190704 ÷ 5493219578125234060 = 0.53208888623796
To be able to make a 4th order polynomial in one unknown out of these four numbers will require that two of them are given a negative sign value...
− 1 and
− 0.53208888623796
Now we can form this polynomial by multiplying these four values together. But first, we have to turn them into linear expressions in one unknown...
X1 = 2.8793852415718
Subtract 2.8793852415718 from both sides of the equal sign...
X1 − 2.8793852415718 = 2.8793852415718 − 2.8793852415718
Yields...
(X − 2.8793852415718) = 0
X2 = − 1
Add 1 to both sides of the equal sign...
X2 + 1 = − 1 + 1
Yields...
(X + 1) = 0
X3 = 0.65270364466614
Subtract 0.65270364466614 from both sides of the equal sign...
X3 − 0.65270364466614 = 0.65270364466614 − 0.65270364466614
Yields...
(X − 0.65270364466614) = 0
X4 = − 0.53208888623796
Add 0.53208888623796 to both sides of the equal sign...
X4 + 0.53208888623796 = − 0.53208888623796 + 0.53208888623796
Yields...
(X + 0.53208888623796) = 0
Multiplying these four roots together...
(X − 2.8793852415718) × (X + 1) × (X − 0.65270364466614) × (X + 0.53208888623796) = 0
Yields...
X4 − 2.8793852415718X3 + 1X3 − 0.65270364466614X3 + 0.53208888623796X3 Ê
Ä − 2.8793852415718X2 + 1.8793852415718X2 − 1.532088886238X2 Ê
Ä − 0.65270364466614X2 + 0.53208888623796X2 − 0.34729635533386X2 Ê
Ä + 1.8793852415718X1 − 1.532088886238X1 + 1X1 − 0.34729635533386X1 + 1 = 0
Simplifying further, yields a 4th order polynomial in one unknown...
X4 -2X3 -3X2 + 1X1 + 1 = 0
The Expanded Euclidean Algorithm arises from the Infinite Range of Golden Ratios...